In percent of change problems, some quantity either increases or decreases from its original number; we must find the percent of change from the original number to the new number. We'll look at a problem and solve it using two different methods.
 
 

Method 1: A pure algebraic approach

1. The unknown in this problem is the percent of decrease. A percent of decrease is the percentage of the original price that was deducted from the original price to obtain the sale price. We could write a "word equation" for this process like this:

   original price - (percent of decrease)(original price) = sale price

   
   
   

   Since the percent of decrease is the unknown in this problem, we'll call the percent of decrease x%.
   
   Here's what we know so far:
   

   The original price was $200

   The sale price was $170

   The percent of increase was x%
    
    

2. Putting this information in our word equation gives us an algebraic equation:

   200 - (x%)(200) = 170

   What do we do with the percent sign in this equation? "Percent" means "out of 100", so x% means x out of 100, or 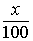. Now we have this:

   200 - 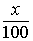(200) = 170

   
   

3. Now we'll solve our equation:

   200 - 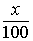(200) = 170

   200 - 2x = 170

   -2x = -30

   x = 15

   So the percent of decrease is 15%

 
 

Method 2: A method involving pure arithmetic.

If we know the original price and the sale price, we can find the percent of decrease in two steps:

1. Find the amount of decrease
2. Divide the amount of decrease by the original amount

This will give us the percent of decrease (written as a decimal number). We can then write the decimal as an equivalent percent to find our final answer.
 
 

Let's use method 2 on the same problem:

Frank bought a new television set on sale. If the TV's original price was $200 and Frank paid $170 for the TV, what was the percent of decrease?

First, let's find the amount of decrease:

Amount of decrease = 200 - 170 = 30 So the price decreased by $30.
 
 

Next, we'll find the percent of decrease, and rewrite the decimal answer as a percent:

Percent of decrease = 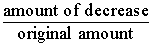 = = 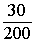.15 = 15% So the percent of decrease is 15%
 
 

Before we leave the problem, let's check our answer:

Original price = $200

Price reduced by 15% of original price = 200(.15) = $30

Sale price would then be = $200 - $30 = $170

Our answer checks. We're done!